GATE Civil 2022 Set 1 | GA Question: 5

Question

In the following diagram, the point $\text{R}$ is the center of the circle. The lines $\text{PQ}$ and $\text{ZV}$ are tangential to the circle. The relation among the areas of the squares, $\text{PXWR, RUVZ}$ and $\text{SPQT}$ is

 

• A. Area of $\text{SPQT}$ = Area of $\text{RUVZ}$ – Area of $\text{PXWR}$
• B. Area of $\text{SPQT}$ = Area of $\text{PXWR}$ –  Area of $\text{RUVZ}$
• C. Area of $\text{PXWR}$ = Area of $\text{SPQT}$ – Area of $\text{RUVZ}$
• D. Area of $\text{PXWR}$ = Area of $\text{RUVZ}$ – Area of $\text{SPQT}$

Answer 1

Let the side of the square $\square \text{SPQT and } \square\text{RUVZ}$ be $a\;\text{cm and }b\;\text{cm}$ respectively.

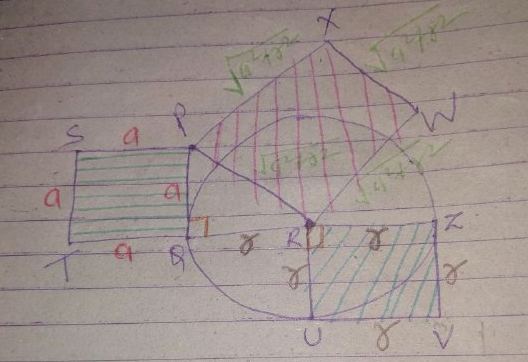
 The triangle $\triangle \text{PQR}$ is a right-angle triangle. So we can apply the Pythagorean theorem.

${\color{Green}{\boxed{\text{(Hypotenuse)}^{2} = \text{(Perpendicular)}^{2} + \text{(Base)}^{2}}}}$

$\Rightarrow (\text{PR})^{2} = (\text{PQ})^{2} + (\text{QR})^{2}$

$\Rightarrow (\text{PR})^{2} = a^{2} + r^{2}$

$\Rightarrow \boxed{\text{PR} = \sqrt{a^{2} + r^{2}}}$

Now, we can easily calculate the area of  the squares, $\square \text{PXWR}, \square \text{RUVZ} \;\text{and}\; \square \text{SPQT}:$

• Area of $\square \text{SPQT} = a^{2}\;\text{cm}^{2}$
• Area of $\square \text{RUVZ} = r^{2}\;\text{cm}^{2}$
• Area of $\square \text{PXWR} = (a^{2} + r^{2})\;\text{cm}^{2}$

We can clearly see, $\text{Area of}\; \square \text{PXWR =  Area of}\; \square \text{SPQT +  Area of}\; \square \text{RUVZ}$

$\Rightarrow {\color{Blue}{\boxed{\text{Area of}\; \square \text{SPQT =  Area of}\; \square \text{PXWR -  Area of}\; \square \text{RUVZ}}}}$

Correct Answer $:\text{B}$