Let the side length of an equilateral triangle be $’x’\;\text{cm}.$
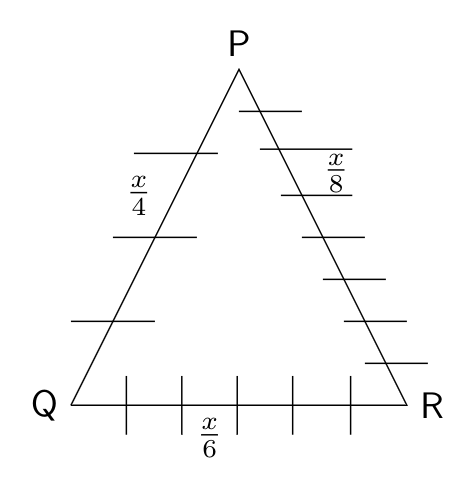
As mentioned in the question, the length of each subdivided part in $\text{cm}$ is an integer. So, the side length must be the LCM of $(4,6,8) \implies x = 24\;\text{cm}.$
Now, the area of an equilateral triangle of side $x\;\text{cm} = \dfrac{\sqrt{3}}{4}\; x^{2} = \dfrac{\sqrt{3}}{4} \;24^{2} = 144\;\sqrt{3}\;\text{cm}^{2}.$
So, the correct answer is $(D).$